Some graphs having mathematical meanings show some beauty, either mathematical one or aesthetic one or both. To appreciate mathematical beauty in a graph you may need some or deep mathematical knowledge. To appreciate purely aesthetic beauty in a graph you do not have mathematical knowledge but should have some sense of art beauty. Most Westerners may find beauty in symmetric graphs while most Oriental people, especially Chinese and Japanese (maybe some other countries and regions too) people can find beauty in asymmetric graphs more than in symmetric ones. Chinese painting theory often refer to the beauty derived from Contrast - which is usually asymmetric. Let's see some examples.
"
From: What is the most aesthetically beautiful graph/surface in mathematics, topology, etc.?
https://www.quora.com/What-is-the-most-aesthetically-beautiful-graph-surface-in-mathematics-topology-etc
For fun or to create something of beauty.
The sine function is valuable tool in mathematics and engineering. Its graph is beautiful and an ideal function to use to compose other playful and beautiful functions. Below are two examples.
In the first composition, 1.5sin(.2x²), the augument of the sine is a flattened parabola which gives the graph symmetry and decreases the "speed" at which the sine goes through its cycle. The multiplication of this by the constant 1.5 gives the function a bit more height compared to the height of the sine function.
In this second composition, the height will continue to grow because the natural log function, seen as ln(x+4), continues to grow and here multiplies the height of the sine.
The curve is shifted to the left by the linear function x+4 seen
as the arguments of the natural log and the sine.
From: Composition of Functions
http://www.mathnstuff.com/math/spoken/here/2class/300/fx/comp.htm
"
The first simple sin (x) function graph is too monotonous - symmetrical and same frequency (no Contrast of Dense and Rare) and the same size and pattern repeat. The second one is still symmetric but shows Contrast of Dense and Rare though not distinctive. The third graph shows aesthetic beauty in the Oriental way - not symmetrical, showing Contrasts of Dense and Rare and Small and Large. It also indicates direction and movement. These three remind us of wave of course.
http://math2.org/math/integrals/more/gammafun.htm
"
My comment
Very open outwardly while showing some focuses or dead end inwardly at different locations quite irregularly. Quite beautiful in the Oriental way. Not totally closed but show some Contrast of openness and "closedness".
"
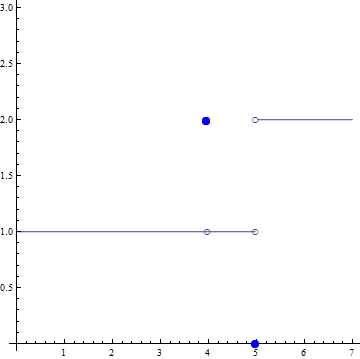
https://www.slader.com/discussion/question/sketch-the-graph-of-a-function-f-that-is-continuous-except-for-the-stated-discontinuity-removable-di/#
"
My comment
This graph does not show "Jump Discontinuity" distinctively at least to me. The positions of two kind of dots (blue and white) and the long and short straight lines show both contrast as well as symmetry. This exhibits spaces partly closed - openly partitioned. So I can point out the following contrasts, at least.
1) Dots and Lines2) Dots - Blue ad white
3) Straight lines - long and short
4) Open and closed
5) Continuous and discontinuous
6) Contrast and symmetry
One more thing, which may be the most important contrast in Chinese paintings
7) Void (虚)and Real(实)
The two Real (can see) lines are horizontal. But when you see the upward jumping discontinuity part you can "feel" or even see in your mind a vertical line. This unseen (Void) vertical line is contrasting the two horizontal real lines. Also we can "feel" or even see the partly closed - openly partitioned spaces (Void).
This is a very attractive graph for me. We cannot neglect the Real x-axis and y-axis to see this graph (also all the other graphs shown in this post). Generally curves are more beautiful than straight line, But the straight line more beautiful than acute angles and spikes. The latter two make people feel fearful and irritated.
Jump Discontinuity - 2
"
Figure 3.4. A function with discontinuities at x=−5, x=−2, x=−1 and x=4.
https://www.sfu.ca/math-coursenotes/Math%20157%20Course%20Notes/sec_ContinuityIVT.html
"
My comment
Now the curves are added to the straight lines. This reminds us of Miro panting. Dots and lines. Curves are more beautiful than straight lines in this graph too. But straight lines act a factor of Contrast of curves and straight lines.
-------
The beauty of Chinese paintings derives from largely countless combinations of these Contrasts. The other important factor contributing to the beauty of Chinese paintings is composition, which is however not just for Chinese paintings but paintings in general.
This may continue as I have found many attractive math graphs.
AAG

No comments:
Post a Comment